Challenge yourself with the most difficult problem in PISA

Asian countries, including Vietnam, far surpass other countries in the PISA exam organized by OECD. Can you solve the most difficult ranking problem in this exam?

>>>Hanging up the "million" prize for the decade problem

Question

A revolving door consists of 3 doors capable of rotating in a circular room. The diameter of this room is 2 meters (200cm). 3 doors divided the room into 3 parts with equal area. The following is a door diagram at different locations, when viewed from the upper vertical corner:

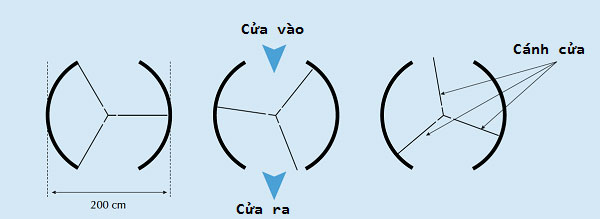
2 parts of the door (break section) are equal in size. If the doors and entrances are too big, the doors will not be able to separate the space; a stream of air can go straight through two doors, from outside the building into the building (causing unwanted rise / fall in the house temperature). Look at the picture below to visualize the path of the air flow in case the size of the two doors is too large.

So, what is the maximum length of the dashed curve of each entry / exit section, so that the air cannot go straight from the entrance to the entrance and vice versa?

Answer

Maximum score: The answer is 103 to 105. The accepted answer is calculated by the formula equal to 1/6 of the circumference of the circle around the room. The answer with 100 is also acceptable, if the candidate counts pi = 3. If the answer is 100 and no explanation is given, the answer will not be graded (because the candidate may have guessed the answer). words equal the length of the door, ie the radius of the circle).

No points: All other answers. Exclude answer 209 (equivalent to the total size of both gates, instead of each door as required).

Explain

To get the maximum score, the competitor must answer that the maximum size of the door is 1/6 of the circumference of the circle, calculated exactly in centimeters.

According to the chart above, the air will move from the outside through the entrance to the exit door if the wall between the door and the door is shorter than the circumference of the two doors in a row. Since each part of the wall is about one-third the size of the perimeter of the room, and has two doors equivalent to two-thirds of the circumference, so the total size of doors and entrances must be less than 1 - 2/3 = 1/3 perimeter. Since doors and entrances are the same size, each door will have to be smaller (1/3) / 2 = 1/6 perimeter of the room.

The above question is one of the most difficult questions in the PISA exam , which is in the upper part of the hardest rank (Grade 6). This question requires candidates to have good thinking about geometry (space and shape) . Also because of the difficulty of this question, the competitor can only get the maximum score, or no score. Although only basic math thinking is required, this question requires candidates to carefully analyze based on geometric thinking.